
Masahisa Wada
Professor, Graduate School of Agriculture, Kyoto University, Japan
He graduated from the Faculty of Agriculture, Tokyo University of Agriculture and Technology and completed the master’s program at the University of Tokyo’s Graduate School of Agriculture in 1993. He started his career as a research fellow of the Japan Society for the Promotion of Science (JSPS) in 1994 and then joined The University of Tokyo as a research associate. He received his PhD from the University of Tokyo in 1997. From 2000 to 2001, he was a visiting lecturer at the Department of Physics, University of Bristol, UK. He joined Kyoto University as an associate professor in 2014 and then was appointed professor in 2018. His research interests focus on solid-state structure-property relationships of polysaccharides such as cellulose. He received The Cellulose Society of Japan Award for 2011.
Cellulose microcrystals prepared by treating natural cellulose (cellulose I) with acid are slender rods or whiskers of a few nanometers width and are known to have properties such as high strength, high elastic modulus, and low thermal expansion1, 2. It has also long been known that cellulose, an antimagnetic material, has magnetic anisotropy3 and various studies have been reported on the response of microcrystals of cellulose I to a strong magnetic field4-10. On the other hand, there have been no studies on the magnetic field response of cellulose II11, the crystalline form of regenerated cellulose fibers such as rayon. Therefore, this article summarizes the magnetic field orientation behavior of cellulose I microcrystals and introduces our recent efforts on the enzymatic synthesis of cellulose II microcrystals and their three-dimensional orientation using a magnetic field12.
When a static magnetic field B is applied to a diamagnetic material, the obtained magnetic energy E is expressed as
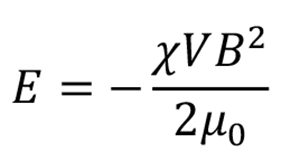
where, χ and V are the anisotropic magnetic susceptibility and volume of the material, and μ0 is the magnetic permeability of the vacuum. However, for materials with anisotropy (magnetic anisotropy), such as crystals, the magnetic energy obtained depends on the orientation to the magnetic field. When the obtained magnetic energy is sufficiently greater than other competing energies, such as thermal energy, the crystal will be oriented in an energetically stable direction.
The magnetic anisotropy of a crystal is represented by the second-order susceptibility tensor χ. Biaxial crystals such as orthorhombic, monoclinic, and triclinic crystals have three different principal values, χ3 < χ2 < χ1 < 0. They are energetically most stable when the largest χ1 axis (easy magnetization axis) is oriented in the direction of the magnetic field. In other words, under a static magnetic field, the crystals are oriented with the χ1 axis aligned in the magnetic field direction.
In nature, cellulose exists as crystalline microfibrils mostly in plant cell walls. Because of their width on the order of nanometers, they have sometimes been referred to as nanofibers in recent years. Cellulose microfibrils are more than several micrometers long but can be cleaved into short fibers by acid hydrolysis, and the acid hydrolysates are called cellulose microcrystals or nanocrystals (Fig. 1a). Three major methods are used to align cellulose microcrystals. One is shear-induced orientation in a fluid flow field, in which a fluid flow field is used to prepare uniaxially oriented films from suspensions of microcrystals14. The second is electric field-induced orientation, in which an alternating electric field is applied to orient the long axis of suspended microcrystals in parallel to the electric field direction15, 16. The last is magnetic field-induced orientation. Unlike fluid flow and electric fields, magnetic fields act through space. Therefore, it is possible to orient a bulk sample by applying a magnetic field. In cellulose microcrystals, the χ3 axis (hard magnetization axis) coincides with the long axis (fiber axis) of the microcrystal, and the values χ1 and χ2 are nearly equal (χ1 ≈ χ2)10. Therefore, the long axis of the microcrystal is oriented perpendicular to the magnetic field4-10.
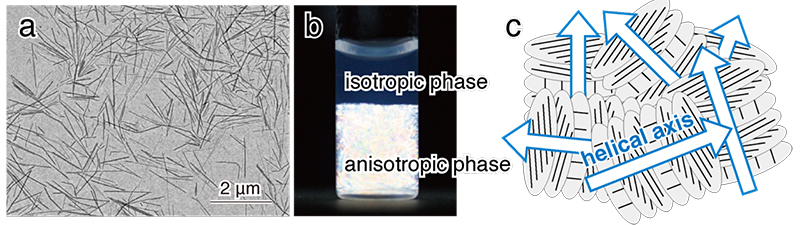
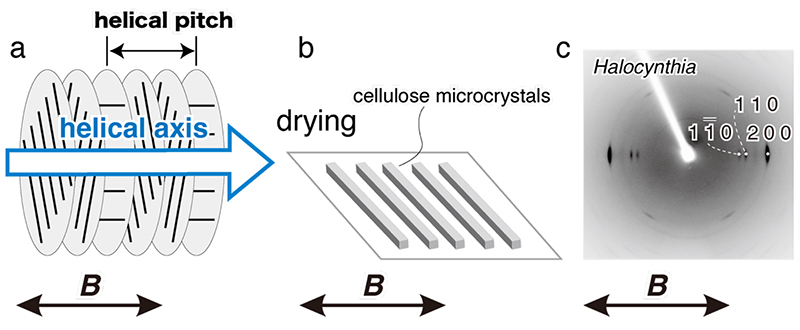
For cellulose microcrystals to be oriented in a magnetic field, the microcrystals must be (1) large in volume and (2) dispersed without aggregation in suspension. In cell walls of green algae and tests of the tunicate Halocynthia roretzi, cellulose crystals have large size. When these celluloses are hydrolyzed with sulfuric acid, a negative charge (-SO3-) is introduced to the surface of the microcrystals, and a suspension of microcrystals with excellent dispersibility can be prepared. It is known that the cellulose microcrystal suspension separates into isotropic and anisotropic phases when the cellulose concentration exceeds a certain critical level (Fig. 1b), and in the anisotropic phase (liquid crystal phase), the microcrystals form a liquid crystal displaying chiral nematic orientation (Fig. 1c)17, 18. When a static magnetic field is applied to the liquid crystal phase, the individual domains become a large monodomain and the helical axes align parallel to the magnetic field (Fig. 2a)6, 7. This is because the fiber axis aligns perpendicular to the magnetic field and is energetically stable. X-ray diffraction diagrams of the film prepared by casting the cellulose microcrystal suspension under a horizontal magnetic field (8 T) showed that the fiber axis was oriented perpendicular to the magnetic field direction (Fig. 2c). This result indicates that the free rotation of the fiber axis around the magnetic field axis (helical axis) was restricted by drying (Fig. 2b), resulting in uniaxial orientation of the microcrystals.
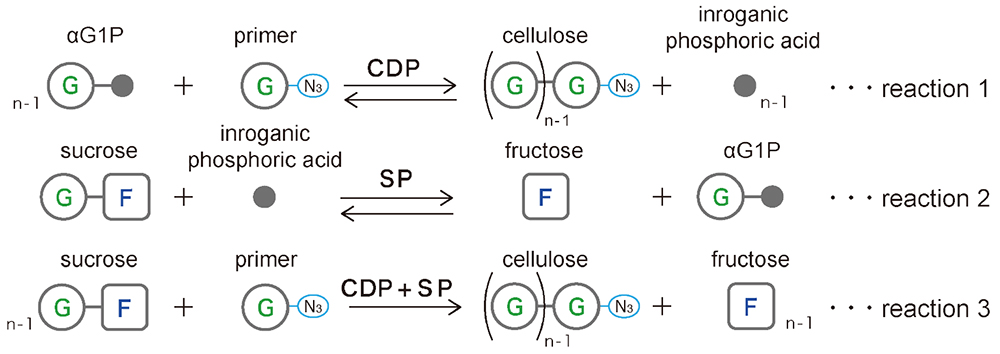
Glycans can be synthesized using the reverse reaction of phosphorylase19. Synthesis using cellodextrin phosphorylase (CDP) with glucose as a primer and α-D-glucose 1-phosphate (αG1P) as a donor yields highly crystalline cellulose II microcrystals composed of cellulose oligomers (DP ≈ 9) (Fig. 3 Reaction 1)20, 21. However, these microcrystals do not have surface charge, which leads to poor dispersion and aggregation in suspension. Therefore, we synthesized microcrystals of cellulose oligomers with azido groups at the reducing end using 1-azido-1-deoxy-β-D-glucopyranoside as a primer22. In this process, sucrose phosphorylase (SP), which degrades sucrose to fructose and αG1P (a href="#fig_3">Fig. 3, Reaction 2), was used with CDP to synthesize cellulose oligomers from sucrose instead of from αG1P (Fig. 3, Reaction 3)23.
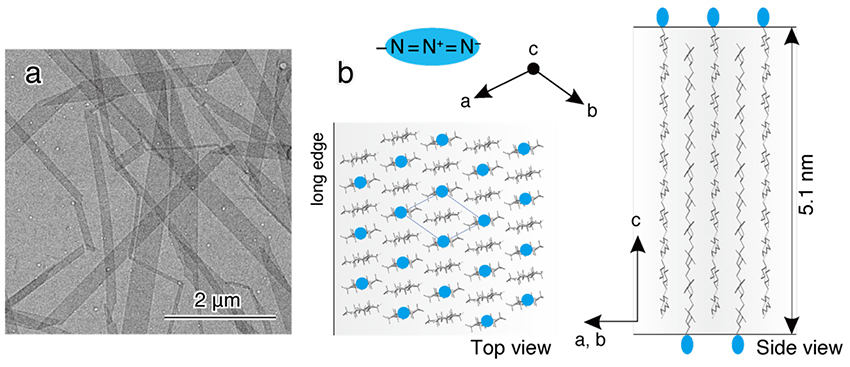
The synthesized product obtained as a white precipitate was dissolved in hot water, then cooled and recrystallized. Recrystallization yielded plate-like cellulose II microcrystals with a width of several hundred nm, a length of several μm, and a thickness of 5 nm, corresponding to the length of the molecular chain (Fig. 4a). The arrangement of cellulose molecular chains in this microcrystal is shown in Figure 4b. The long edge of the microcrystal corresponds to the [1 1 0] direction of cellulose II, thus the crystal growth is in the direction of hydrophobic stacking of the glucose ring. In addition, azido groups are placed on the surface of the microcrystals. Therefore, the suspension of recrystallized microcrystals did not aggregate, and bright birefringence was observed between crossed polarizers. In other words, cellulose II microcrystals with large size and excellent dispersibility, which seem to be capable of magnetic field orientation, were prepared by using CDP.
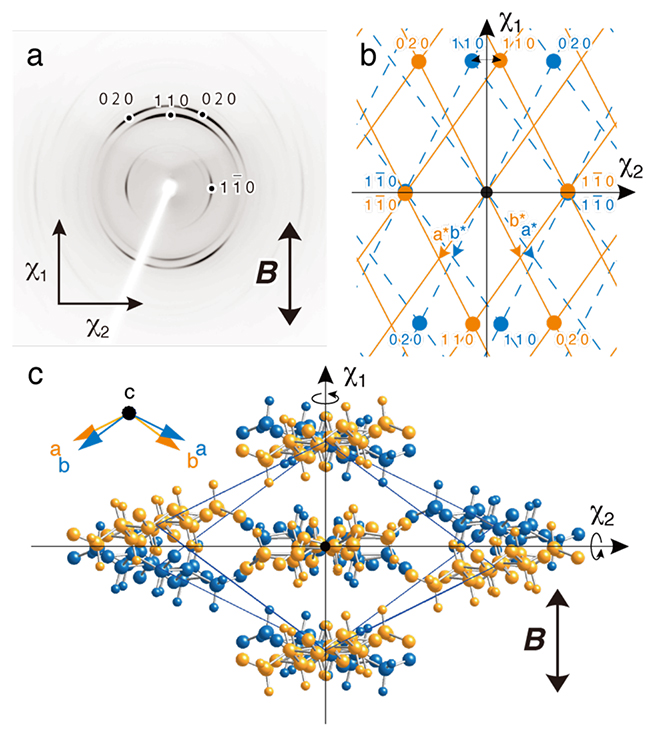
Cellulose II microcrystal suspensions were cast and dried under a horizontal magnetic field of 8 T and a film with three-dimensional orientation of the cellulose microcrystals was obtained. In the diffraction diagram with X-rays aimed perpendicular to the film surface (Fig. 5a), 1 1 0 diffraction appeared in the magnetic field direction and 1 1 0 diffraction appeared perpendicular to the magnetic field direction. This indicates that the χ1 axis and the χ2 axis of cellulose II are approximately in the [1 1 0] and [1 1 0] directions, respectively (Fig. 5b). Although three-dimensional orientation is usually achieved by time-modulated magnetic fields24, 25, this was accomplished by a combination of the χ1 axis orientation in a static magnetic field and a flat-on arrangement of plate-like microcrystals by drying. However, since the magnetization axis has no positive or negative direction, the magnetic energy will be the same even if the crystal is rotated 180° around the magnetization axis. Therefore, the monoclinic cellulose II microcrystals are in twin orientation (Fig. 5c).
Cellulose synthesized by CDP formed plate-like microcrystals of cellulose II, which exhibited a molecular chain arrangement different from that of cellulose I microcrystals. By placing azido groups on the surface of cellulose II microcrystals, a suspension of cellulose II with excellent dispersibility could be prepared, and three-dimensional alignment of the cellulose II microcrystals could be achieved under a static magnetic field. In this study, the strong magnetic field generated by a superconducting magnet was used, but even a magnetic field as small as that of a permanent magnet can be used in combination with a fluid flow field to achieve a high degree of cellulose microcrystal alignment. Moreover, by using a strong magnetic field, it is possible to achieve three-dimensional orientation of microcrystals of polysaccharides and polymers other than cellulose, opening the way for structural analysis and the creation of anisotropic materials.
The author thanks Dr. Ryosuke Kusumi, Forestry and Forest Products Research Institute, for comments on this article.